Continuous Functions That Are Not Differentiable
HOW TO PROVE THAT THE FUNCTION IS NOT DIFFERENTIABLE
About "How to Prove That the Function is Not Differentiable"
How to Prove That the Function is Not Differentiable ?
Here we are going to see how to prove that the function is not differentiable at the given point.
The function is differentiable from the left and right. As in the case of the existence of limits of a function at x0, it follows that
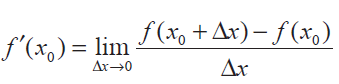
exists if and only if both
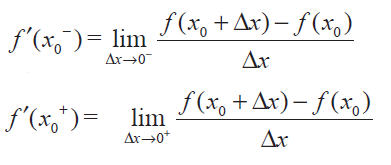
exist and f' (x0-) = f' (x0+)
Hence
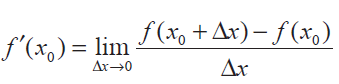
if and only if f' (x0-) = f' (x0+). If any one of the condition fails then f'(x) is not differentiable at x0.
From the above statements, we come to know that if f' (x0-) ≠ f' (x0+), then we may decide that the function is not differentiable at x0.
How to Prove That the Function is Not Differentiable - Examples
Question 1 :
Show that the following functions are not differentiable at the indicated value of x.
(i)
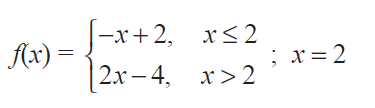
Solution :
f'(2-) = limx->2- [(f(x) - f(2)) / (x - 2)]
= limx->2- [(-x + 2) - (-2 + 2)] / (x - 2)
= limx->2- -(x - 2) / (x - 2)
= limx->2- (-1)
= -1 -----(1)
f'(2+) = limx->2+ [(f(x) - f(2)) / (x - 2)]
= limx->2+ [(2x - 4) - (4 - 4)] / (x - 2)
= limx->2+ 2(x -2) / (x - 2)
= 2 -----(2)
f'(2 - ) ≠ f'(2 + )
Hence the given function is not differentiable at the point x = 2.
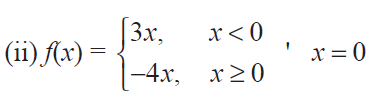
Solution :
f'(0-) = limx->0- [(f(x) - f(0)) / (x - 0)]
= limx->0- [(3x) - 0] / (x - 0)
= limx->0- (3x/x)
= limx->0- 3
= 3 -----(1)
f'(0+) = limx->0+ [(f(x) - f(0)) / (x - 0)]
= limx->0+ [(-4x) - 0] / (x - 0)
= limx->0+ (-4x/x)
= limx->0+ -4
= -4 -----(2)
f'(0 - ) ≠ f'(0 + )
Hence the given function is not differentiable at the point x = 0.
Question 2 :
The graph of f is shown below. State with reasons that x values (the numbers), at which f is not differentiable.
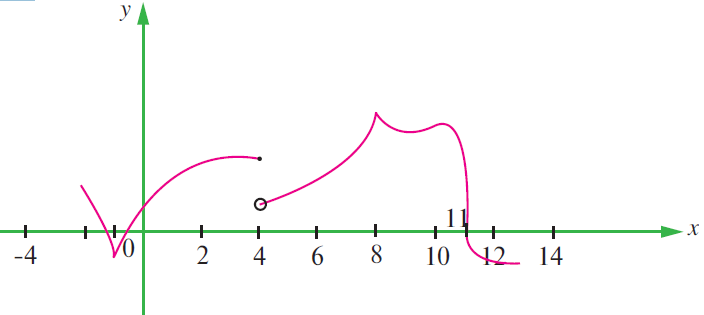
Solution :
If a function is continuous at a point, then it is not necessary that the function is differentiable at that point.
A function f is not differentiable at a point x0 belonging to the domain of f if one of the following situations holds:
(i) f has a vertical tangent at x0.
(ii) The graph of f comes to a point at x0 (either a sharp edge ∨ or a sharp peak ∧ )
(iii) f is discontinuous at x0.

At x = 1 and x = 8, we get vertical tangent (or) sharp edge and sharp peak. So it is not differentiable at x = 1 and 8.
At x = 4, we hjave a hole. Hence it is not continuous at x = 4.
At x = 11, we have perpendicular tangent. So it is not differentiable at x = 11.
Question 3 :
If f(x) = |x + 100| + x2, test whether f'(-100) exists.
Solution :
f'(-100-) = limx->-100- [(f(x) - f(-100)) / (x - (-100))]
= limx->-100- [(-(x + 100)) + x2) - 1002] / (x + 100)
= limx->-100- [(-(x + 100)) + (x2 - 1002)] / (x + 100)
= limx->-100- [(-(x + 100)) + (x + 100) (x -100)] / (x + 100)
= limx->-100- (x + 100)) [-1 + (x -100)] / (x + 100)
= [-1 + (-100 -100)]
= -201 --------(1)
f'(-100+) = limx->-100+ [(f(x) - f(-100)) / (x - (-100))]
= limx->-100- [(x + 100)) + x2) - 1002] / (x + 100)
= limx->-100- [(x + 100)) + (x2 - 1002)] / (x + 100)
= limx->-100- [(x + 100)) + (x + 100) (x -100)] / (x + 100)
= limx->-100- (x + 100)) [1 + (x -100)] / (x + 100)
= limx->-100- (x - 99)
= -199 --------(2)
Hence f'(-100) does not exists.
Question 4 :
Examine the differentiability of functions in R by drawing the diagrams.
(i) | sin x |
Solution :
Graph of y = sin x
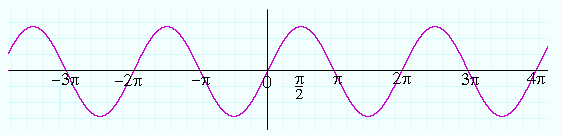
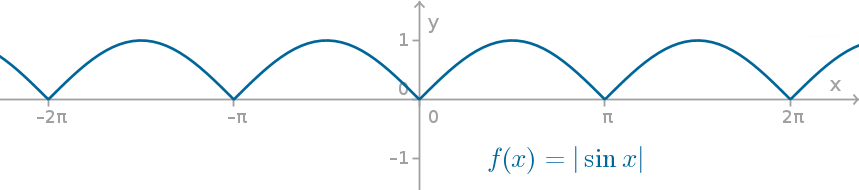
There is vertical tangent for nπ. Hence it is not differentiable at x =nπ, n ∈ z
(ii) | cos x|
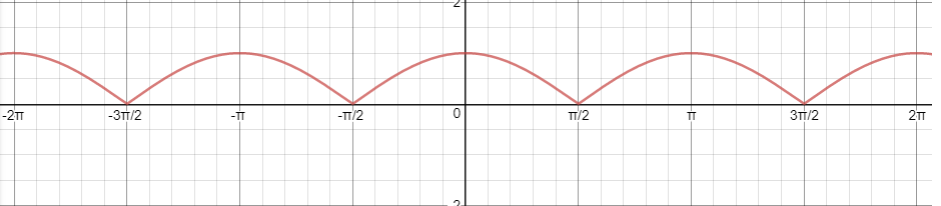
Solution :
There is vertical tangent for (2n + 1)( π/2) . Hence it is not differentiable at x = (2n + 1)( π/2), n ∈ z

After having gone through the stuff given above, we hope that the students would have understood, " How to Prove That the Function is Not Differentiable"
Apart from the stuff given in " How to Prove That the Function is Not Differentiable" , if you need any other stuff in math, please use our google custom search here.
Kindly mail your feedback tov4formath@gmail.com
We always appreciate your feedback.
© All rights reserved. onlinemath4all.com
Source: https://www.onlinemath4all.com/how-to-prove-that-the-function-is-not-differentiable.html
0 Response to "Continuous Functions That Are Not Differentiable"
Post a Comment